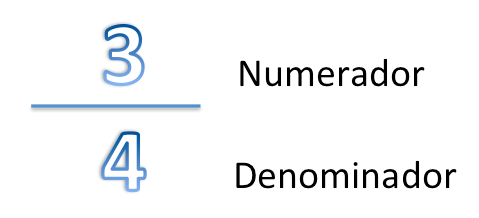
Método de Evaluación de Límites. Indeterminaciones IV
A parte de las indeterminaciones estudiadas, existen otras de diversa índole que aparecen en la resolución de ciertos límites que sobrepasan la forma tradicional de resolución. Veremos varios de esos límites esta página.
Ejemplos.
Nótese que en todos los ejemplos ilustrados la variable x tiende a un número finito o a cero. Esta es una parte de todas las formas de límite que existen. En realidad también la variable x puede tender a . Estos ejemplos los veremos en la próxima lección.