Método de Resolución de Límites. División por la mayor potencia II

Vamos a seguir profundizando en el alcance de este método de resolución. Nótese que en la lección anterior se resolvieron varios ejercicios donde se aplicó el método de dividir por la mayor potencia.

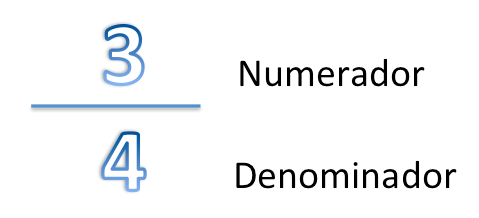
Resumiendo su metodología:

Encontrar la variable que tiene mayor potencia en toda la función.
Dividir cada término de la función entre la mayor potencia.
Una vez realizados todo estos pasos proceder a determinar el límite.

Ejemplos:

$f left parenthesis x right parenthesis equals space fraction numerator 7 x squared plus 4 x plus 5 over denominator 2 x squared plus 6 x plus 9 end fraction equals left parenthesis 1 right parenthesis space space fraction numerator 7 x squared plus 4 x plus 5 over denominator 2 x squared plus 6 x plus 9 end fraction equals space open parentheses x squared over x squared close parentheses space fraction numerator 7 x squared plus 4 x plus 5 over denominator 2 x squared plus 6 x plus 9 end fraction equals open parentheses fraction numerator 1 over denominator begin display style x squared over x squared end style end fraction close parentheses fraction numerator 7 x squared plus 4 x plus 5 over denominator 2 x squared plus 6 x plus 9 end fraction equals fraction numerator begin display style fraction numerator 7 x squared plus 4 x plus 5 over denominator x squared end fraction end style over denominator begin display style fraction numerator 2 x squared plus 6 x plus 9 over denominator x squared end fraction end style end fraction equals fraction numerator begin display style fraction numerator 7 x squared over denominator x squared end fraction end style plus begin display style fraction numerator 4 x over denominator x squared end fraction end style plus begin display style 5 over x squared end style over denominator begin display style fraction numerator 2 x squared over denominator x squared end fraction end style plus begin display style fraction numerator 6 x over denominator x squared end fraction end style plus begin display style 9 over x squared end style end fraction equals fraction numerator 7 plus 4 over x plus 5 over x squared over denominator 2 plus 6 over x plus 9 over x squared end fraction stack l i m with x rightwards arrow infinity below space fraction numerator 7 plus 4 over x plus 5 over x squared over denominator 2 plus 6 over x plus 9 over x squared end fraction equals fraction numerator 7 plus 0 plus 0 over denominator 2 plus 0 plus 0 end fraction equals 7 over 2 g left parenthesis x right parenthesis equals fraction numerator x to the power of 7 plus x to the power of 6 plus 2 x to the power of 5 over denominator x to the power of 7 plus x to the power of 5 plus 3 x cubed plus 4 x to the power of 6 plus 2 x squared end fraction equals left parenthesis 1 right parenthesis equals open parentheses x to the power of 7 over x to the power of 7 close parentheses fraction numerator x to the power of 7 plus x to the power of 6 plus 2 x to the power of 5 over denominator x to the power of 7 plus x to the power of 5 plus 3 x cubed plus 4 x to the power of 6 plus 2 x squared end fraction equals equals open parentheses fraction numerator 1 over denominator begin display style x to the power of 7 over x to the power of 7 end style end fraction close parentheses fraction numerator x to the power of 7 plus x to the power of 6 plus 2 x to the power of 5 over denominator x to the power of 7 plus x to the power of 5 plus 3 x cubed plus 4 x to the power of 6 plus 2 x squared end fraction equals fraction numerator begin display style fraction numerator x to the power of 7 plus x to the power of 6 plus 2 x to the power of 5 over denominator x to the power of 7 end fraction end style over denominator begin display style fraction numerator x to the power of 7 plus x to the power of 5 plus 3 x cubed plus 4 x to the power of 6 plus 2 x squared over denominator x to the power of 7 end fraction end style end fraction equals fraction numerator begin display style x to the power of 7 over x to the power of 7 end style plus begin display style x to the power of 6 over x to the power of 7 end style plus begin display style fraction numerator 2 x to the power of 5 over denominator x to the power of 7 end fraction end style over denominator begin display style x to the power of 7 over x to the power of 7 end style plus begin display style x to the power of 5 over x to the power of 7 end style plus begin display style fraction numerator 3 x cubed over denominator x to the power of 7 end fraction end style plus begin display style fraction numerator 4 x to the power of 6 over denominator x to the power of 7 end fraction end style plus begin display style fraction numerator 2 x squared over denominator x to the power of 7 end fraction end style end fraction equals equals fraction numerator begin display style 1 plus 1 over x plus 2 over x squared end style over denominator begin display style 1 plus 1 over x squared plus 3 over x to the power of 4 plus 4 over x plus 2 over x to the power of 5 end style end fraction stack l i m with x rightwards arrow infinity below space fraction numerator begin display style 1 plus 1 over x plus 2 over x squared end style over denominator begin display style 1 plus 1 over x squared plus 3 over x to the power of 4 plus 4 over x plus 2 over x to the power of 5 end style end fraction equals space space fraction numerator begin display style 1 plus 0 plus 0 end style over denominator begin display style 1 plus 0 plus 0 plus 0 plus 0 end style end fraction$

Como usted puede apreciar, este procedimiento resuelto detalladamente conlleva mucho tiempo y esfuerzo, por ello es que se omiten pasos los cuales se asume "el estudiante ya conoce". ¿Qué pasos considera que no son absolutamente necesarios?