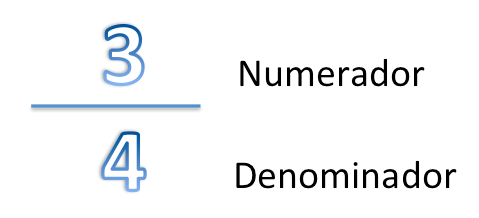
Método de Resolución de Límites. División por la mayor potencia

En esta lección vamos a tener ejemplos de otro método de resolución de límites. Este método consiste en dividir por la mayor potencia que haya en f(x) o en g(x). Se utiliza mucho si la variable x tiende al infinito.

Ejemplos.

$stack l i m with x rightwards arrow infinity below space fraction numerator 2 x cubed plus 3 over denominator 3 x cubed plus 6 end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 2 begin display style x cubed over x cubed end style plus begin display style 3 over x cubed end style over denominator 3 begin display style x cubed over x cubed end style plus begin display style 6 over x cubed end style end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 2 plus begin display style 3 over x cubed end style over denominator 3 plus begin display style 6 over x cubed end style end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 2 plus begin display style 0 end style over denominator 3 plus 0 end fraction equals 2 over 3 stack l i m with x rightwards arrow infinity below space fraction numerator 2 x squared plus 7 x over denominator 4 x to the power of 4 plus 2 x end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 2 begin display style x squared over x to the power of 4 end style plus begin display style fraction numerator 7 x over denominator x to the power of 4 end fraction end style over denominator 4 begin display style x to the power of 4 over x to the power of 4 end style plus begin display style fraction numerator 2 x over denominator x to the power of 4 end fraction end style end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator begin display style 2 over x squared end style plus begin display style 7 over x cubed end style over denominator 4 plus begin display style 6 over x cubed end style end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 0 plus begin display style 0 end style over denominator 4 plus 0 end fraction equals 0 over 4 equals 0 stack l i m with x rightwards arrow infinity below space fraction numerator 3 x to the power of 5 plus 2 x over denominator x to the power of 4 plus x end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 3 begin display style x to the power of 5 over x to the power of 5 end style plus begin display style fraction numerator 2 x over denominator x to the power of 5 end fraction end style over denominator begin display style x to the power of 4 over x to the power of 5 end style plus begin display style x over x to the power of 5 end style end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator begin display style 3 plus 2 over x to the power of 4 end style over denominator begin display style 1 over x end style plus begin display style 1 over x to the power of 4 end style end fraction equals stack l i m with x rightwards arrow infinity below space fraction numerator 3 plus begin display style 0 end style over denominator 0 plus 0 end fraction equals 3 over 0 equals infinity$