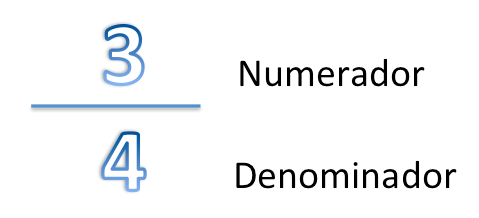
Propiedades fundamentales del límite de una función
En la presente lección abordaremos las propiedades del limite de una función de variable real. Ninguna propiedad va a ser demostrada en este curso. Si el alumno desea conocer más del tema puede visitar las referencias bibliográficas al final del curso.
Propiedades del limite de una función.
1- Si la función f(x)= c, donde c es una constante y la variable x pertenece al campo numérico de los reales, entonces . Es decir, el límite de una función constante es la propia constante c.
2- Si existen los limites finitos de entonces existen también el límite de la suma de ambas funciones y el límite de la multiplicación de ambas funciones.
3- Si es distinto de cero entonces :
.
4- Si existe el limite , entonces para cualquier número real c existe el límite
.
5- Si la función f(x) se encuentra entre dos funciones distintas que la acotan superior en inferiormente, y estas dos funciones distintas tienen el mismo límite cuyo número denominaremos como c, entonces el límite de la función f(x) será exactamente el número c.