Variables que tienden al infinito II
En este epígrafe vamos a poner más ejemplos de límites de funciones cuyas variables tienden al infinito. Antes de ello vamos a exponer un método de resolución de límites muy eficaz y sencillo, y se postulan como teoremas de limites infinitos en el caso de una división cualquiera de dos polinomios cualquiera.
Teorema de Limites Infinitos.
Sean f(x) y g(x) dos polinomios cualquiera de grado n y m respectivamente, sus variables tienden al infinito si:
- El grado n de f(x) es mayor que el de g(x), entonces el resultado del límite será infinito.
- El grado de f(x) es menor que el de g(x), entonces el resultado del límite será cero.
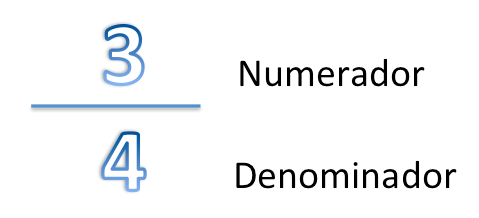
- El grado de f(x) es igual al de g(x), entonces el resultado del límite será la división de los coeficientes de los grados n y m, en ese orden.
Ejemplos.